

In addition it plots the ARL curve for mean or sigma shifts. It shows where to place the control limits for different in-control ARLs. Briefly, a confidence interval is a way of estimating a population parameter that provides an interval of the parameter rather than a. Refer to the Sample Size Calculator for Proportions for a more detailed explanation of confidence intervals and levels. Then, use another column (next to the previous one) that labeled "(X - Xbar)^2", where you square the cells from the column to the left. Calculator Xbar and S Charts 4+ Francisco Aparisi Designed for iPad Free Screenshots iPad iPhone This application computes the ARL for Xbar, S and combined Xbar-S control charts for different mean and sigma shifts. The calculator also provides a table of confidence intervals for various confidence levels.
#Xbar and s calculator how to#
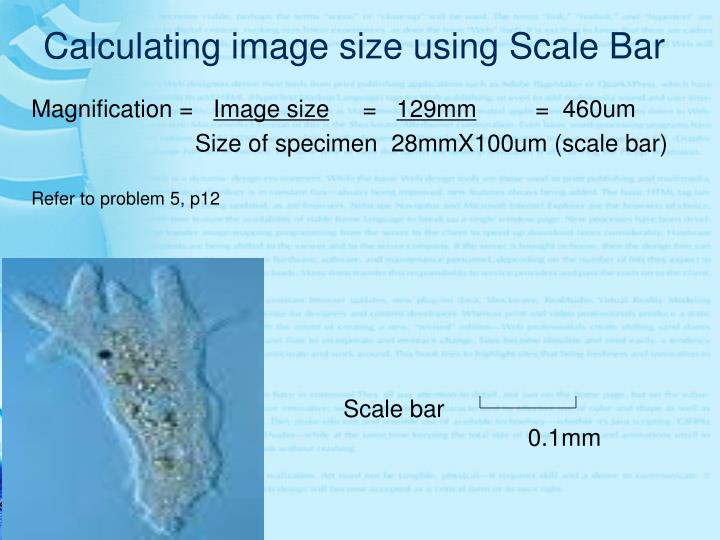
How to calculate sum of squares in Excel? You probably should put your data in a column and label that column as "X", then compute the average for the sample, and create of column labeled "X - Xbar" which computes the value of a corresponding cell minus the average. Using Excel to Compute the Sum of Squares The idea of sum of squares also extends to linear regression, where the regression sum of squares and the residual sum of squares determines the percentage of variation that is explained by the model. X-Bar (Sample Mean) Calculator - Statology Apby Zach X-Bar (Sample Mean) Calculator In statistics, x-bar ( x) is a symbol used to represent the sample mean of a dataset. So, you take the sum of squares \(SS\), you divide by the sample size minus 1 (\(n-1\)) and you have the sample variance. Indeed, the sample variance \(s^2\) is computed as: And it is strongly related to the concept of sample variance. Why the idea of the sum of squares \((SS)\) is so useful in Statistics? Well, mostly for one reason: The sum of squares is a strong indication of the level of variability of a sample. Relationship Between Sum of Squares and Sample Variance Mathematically, the formula to define the sum of squares associated to the sample \(\^n (X_i - \bar X)^2 \]
So, starting from the beginning, the sum of squares usually refers to the sum of squared deviations with respect to the mean, for a sample of data. The concept of sum of squares is a very useful one, which appears almost everywhere in Statistics, but people do not often take the time to explain what it is.


 0 kommentar(er)
0 kommentar(er)
